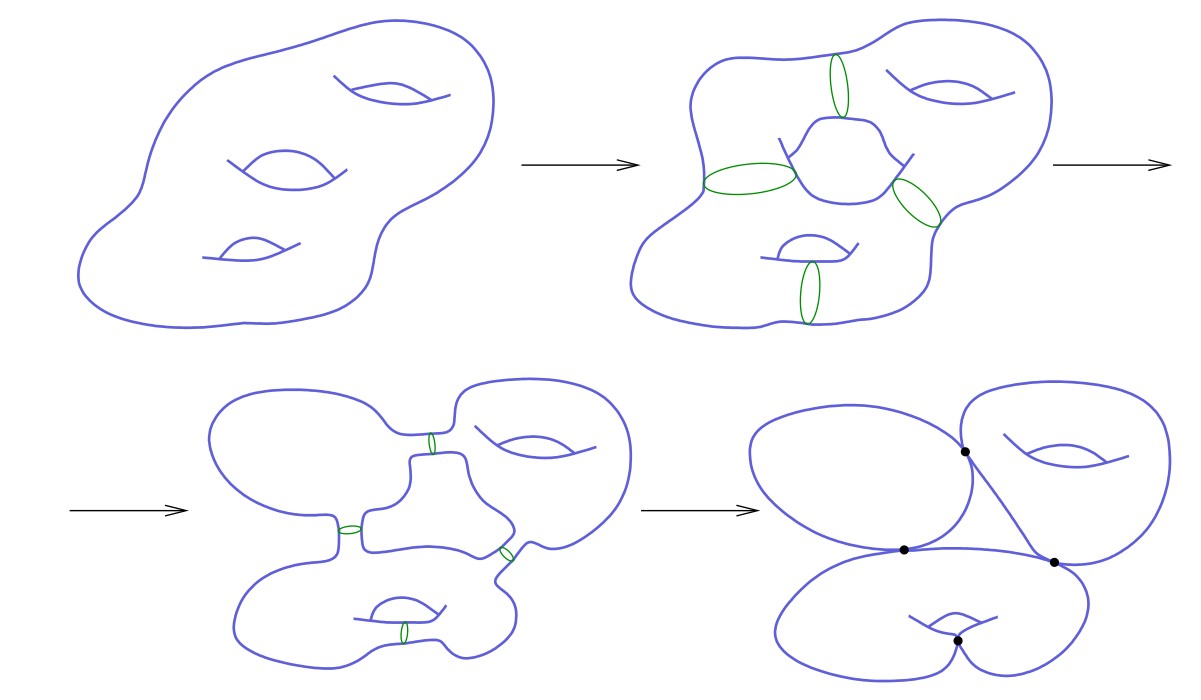
Gromov's Compactness Theorem is a fundamental concept in mathematics, particularly in the field of geometric analysis. It provides a powerful tool for understanding the behavior of sequences of geometric objects, such as Riemannian manifolds, and has far-reaching implications in various areas of mathematics and its applications. Understanding this theorem is crucial for mathematicians and researchers seeking to delve into the intricate world of geometric analysis.
In this article, we will explore six essential facts about Gromov's Compactness Theorem, shedding light on its significance and applications. From its historical background to its practical implications, we will unravel the key aspects of this theorem, providing valuable insights for both aspiring mathematicians and those with a keen interest in the beauty of mathematical theories. So, let's embark on a journey to uncover the fascinating world of Gromov's Compactness Theorem and its profound impact on the realm of mathematics.
Key Takeaways:
- Gromov’s Compactness Theorem helps mathematicians understand how sequences of shapes behave, like a roadmap for exploring the limits and patterns of geometric objects.
- This theorem has transformed the way we study shapes and equations, opening doors to new discoveries in math and even in the real world.
Gromov's Compactness Theorem is a fundamental concept in mathematics.
Gromov's Compactness Theorem is a fundamental concept in mathematics that has far-reaching implications in various fields. It provides a powerful tool for understanding the behavior of sequences of geometric objects and has applications in areas such as differential geometry, topology, and mathematical physics. The theorem was introduced by the renowned mathematician Mikhail Gromov and has since become a cornerstone of modern mathematics.
It plays a crucial role in understanding the behavior of sequences of geometric objects.
Gromov's Compactness Theorem plays a crucial role in understanding the behavior of sequences of geometric objects. It provides a framework for analyzing the limiting behavior of sequences of spaces, such as Riemannian manifolds, metric spaces, and symplectic manifolds. This is particularly valuable in studying the convergence of sequences of solutions to geometric and analytical problems, shedding light on the underlying structures and their properties.
The theorem has wide-ranging applications in differential geometry and topology.
The theorem has wide-ranging applications in differential geometry and topology, offering insights into the global and local properties of geometric spaces. It has been instrumental in the study of moduli spaces, which represent the collection of all geometric structures of a given type on a fixed underlying space. Additionally, the theorem has found applications in the theory of minimal surfaces, isoperimetric inequalities, and the understanding of symplectic and complex structures on manifolds.
Gromov's Compactness Theorem provides a powerful tool for understanding the behavior of sequences of geometric objects.
Gromov's Compactness Theorem provides a powerful tool for understanding the behavior of sequences of geometric objects. By establishing criteria for the existence of limit spaces, the theorem facilitates the analysis of the convergence and compactness of sequences, offering valuable insights into the underlying geometry and topology. This has profound implications in various mathematical disciplines, enabling mathematicians to explore the intricate structures of spaces and their limiting behavior.
It has implications in mathematical physics and the study of partial differential equations.
The theorem has implications in mathematical physics and the study of partial differential equations, providing a framework for understanding the behavior of solutions to geometric and analytical problems. It has been instrumental in the study of geometric flows, which describe the evolution of geometric structures over time, and has contributed to the understanding of the long-term behavior of solutions to partial differential equations arising in mathematical physics.
Gromov's Compactness Theorem has revolutionized the study of geometric and analytical problems.
Gromov's Compactness Theorem has revolutionized the study of geometric and analytical problems, offering a powerful tool for analyzing the limiting behavior of sequences of geometric objects. Its wide-ranging applications have had a profound impact on various branches of mathematics, providing valuable insights into the convergence, compactness, and limiting behavior of spaces. The theorem continues to be a cornerstone of modern mathematics, shaping the way mathematicians approach and understand geometric and analytical problems.
In conclusion, Gromov's Compactness Theorem stands as a testament to the profound impact of mathematical concepts in shaping our understanding of the world around us. Its far-reaching implications and applications underscore the significance of fundamental theorems in advancing the frontiers of knowledge and unlocking new realms of exploration in mathematics and beyond.
Conclusion
In conclusion, Gromov's Compactness Theorem is a powerful tool in the field of mathematical sciences. Its applications extend across various disciplines, from geometry to analysis, providing valuable insights into the behavior of sequences of geometric objects. Understanding the fundamental concepts and implications of this theorem is crucial for mathematicians and researchers seeking to delve deeper into the intricacies of compactness and convergence in mathematical spaces. By grasping the significance of Gromov's Compactness Theorem, scholars can unlock new avenues for exploration and discovery within the realm of mathematical theory and its practical applications.
FAQs
What is the significance of Gromov's Compactness Theorem?Gromov's Compactness Theorem holds immense significance in mathematical sciences, particularly in the study of geometric structures and their convergence properties. It provides a powerful framework for understanding the behavior of sequences of geometric objects within a given space, offering valuable insights into compactness and limit points.
How does Gromov's Compactness Theorem impact mathematical research?Gromov's Compactness Theorem has a profound impact on mathematical research, enabling scholars to explore and analyze the convergence of geometric structures in various mathematical spaces. This theorem serves as a fundamental tool for investigating the compactness of sequences and has far-reaching implications across diverse mathematical disciplines.
Was this page helpful?
Our commitment to delivering trustworthy and engaging content is at the heart of what we do. Each fact on our site is contributed by real users like you, bringing a wealth of diverse insights and information. To ensure the highest standards of accuracy and reliability, our dedicated editors meticulously review each submission. This process guarantees that the facts we share are not only fascinating but also credible. Trust in our commitment to quality and authenticity as you explore and learn with us.